Nylon Calculus: How will the new G League free throw rule change scoring?

The new G League free-throw rule seems like a radical move that fans either love or hate. Will it actually have a meaningful impact on decision-making and scoring?

The G League just announced that this season they will be using a new rule that replaces free-throws with a single shot worth however many points based on the location of the shooting foul. For instance, a 2-point attempt, 3-point attempt and and-one attempt are each still worth their respected 2, 3 and 1 point(s) but it will come down to just a single attempt at the line to try and earn these points.

For the last two minutes of a game, the rules will revert back to normal with multiple free-throws. The league has toyed with trying this in the G League for years and now finally comes to fruition. The goal is to improve game flow and will hopefully shave off roughly six to eight minutes. Improving game flow is an almost unanimous desire among fans but the initial outrage seems to oppose the experiment. Will the value of the free-throws deviate much and are there potential scoring ramifications of this change?

Value of the free throw

Getting to the line is an efficient way to generate points. The expected points per trip to the line (denoted PPS) relative to shooting from other areas on the court is high. We can calculate this from the formula below:

PPS = Point value of FT * Expected # of attempts per trip * League average FT%

The league average free throw percentage of the last five seasons is 76.1 percent. Instead of averaging 1 attempt, 2 attempts and 3 attempts together and assuming the average number of shots per trip to the line is 2, I found the rate at which each attempt is generated to find out the true average number of attempts per trip. Using play-by-play data from the last five seasons, the proportion of and-ones, technicals and flagrants (1-shot trips) are 10 percent of all free throw attempts. 2-point attempts make up 85.3 percent and 3-point attempts make up the remaining 4.7 percent. So the average number of attempts per trip to the line is

0.047 * 3 + 0.853 * 2 + 0.1003 * 1 = 1.95

Essentially we can expect slightly less than 2 attempts on average for a player to take at the line. Thus the PPS of free-throws under the current rule is:

PPS = 1 * 1.95 * 0.761 = 1.48

Netting almost 1 and a half points from the line prompts a sound, efficient strategy of getting to the rim. Now under the new G League rules how will the value of the free-throw change?

(Aside: The data I’m working with is NBA play-by-play, but the conclusion and environment operate the same in the G League.)

Now to find the expected value of going to the line under these new rules lets consider an example. Take James Harden, a career 86 percent free-throw shooter, heading to the line after being fouled shooting a 3. On average, the free throw percentage on a first attempt is lower than the second attempt which in turn is also lower than the 3rd attempt. Parsing play-by-data finds Harden’s free throw percentages of 84.7 percent on the first attempt, 87.7 percent on the second attempt and 91.5 percent on the third attempt. For the current NBA rule, we can assume independence on all three shots and find the expected value as:

EV = 1 * 0.847 + 1 * 0.877 + 1 * 0.915 = 2.64

We expect Harden to walk away with 2.64 points. Under the new rule of the G-League, Harden has an expected value of:

EV = 3 * 0.847 = 2.54

The expected value under the new rule is slightly lower! For 2 and 3-point attempts, the values of each shot and the number of shots ultimately switch, but the probability of hitting the shot(s) changes resulting in differing expected values.

However, the variance increases. To get an idea of this, consider the same example. If we instead wanted to find the probability that Harden walked away from the line with zero points, under normal rules he would have to miss three straight shots with sequential probabilities of 15.3 percent, 12.3 percent and 8.5 percent. That yields a 0.16 percent chance of happening (0.153 * 0.123 * 0.085). Under the new rule with only one shot, he has a 15.3 percent chance of missing that one shot worth three points and instead of walking away with zero points. The same can be calculated for finding the probability that Harden walks away with three points. Hitting all three shots under the normal rule nets him a likelihood of only 68 percent to assimilate all three points. Under the new rule, he has an 84.7 percent chance of gaining all three points. Voila! Variance! The new rule introduces a higher probability of getting all three points but also a higher probability of returning zero points.

Even though the expected value is lower under this new rule, the higher probability of gaining all possible points is a reason some could argue that this new rule could actually help Harden. Good free throw shooters are more likely to net the maximum points at the line each time whereas bad free throw shooters still have low probabilities in both rules.

How does this look for the whole league? Parsing play-by-play data from NBA Stats, I separated free throws to find how the average free throw percentage changes on the first attempt compared to all subsequent attempts. The chart below details this computed over the last five years from the 2014-15 season to last season.

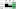
The first attempt whether from a shooting foul on a technical or flagrant, an and-one or a 2-point or 3-point attempt is lower than the adjoining shot. Making the first shot is harder. This is also evidenced on 3-point attempts as well but with much higher percentages. The reason for this being that shooters who get fouled from behind the arc are more likely than not, good free throw shooters. The lower percentage on first attempts also, in turn, contributes to the increase in variance. With a lower probability of making the first attempt, we see higher variance among shot outcomes.

Using the league average free throw percentage on only first attempts and assuming the same rates on how many one-shot, two-shot and three-shot attempts are made we can figure out the PPS for the whole league under the new rule. However, one caveat here is that normal rules are in play for the last two minutes. The updated formula looks like this:

PPS’ = 46/48 * (Average Points Value * Expected # of attempts per trip * League Average FT% on 1st attempts only) + 2/48 * (PPS under normal conditions)

= 46 /48 * (1.95 * 1 * 0.737) + 2/48 * (1.48)

= 1.44

Overall the value of getting to the line decreases ever so slightly from 1.48 to 1.44 for a difference of 0.04. This is a very marginal difference that doesn’t move the needle away from how valuable getting to the line is.

Overall scoring change

Since the value of the free throw slightly changes then it would also make sense there is a marginal difference on total scoring in games. Last year, teams averaged about 23 free throw attempts a game. Translating that to trips we divide by the 1.95 average number of shots per trip to get 11.8 trips per game. Multiplying that by the average PPS of a trip to the line nets on average 17.5 points per game from free throws. This value agrees with the average number of free throws made for teams last year.

Under new rules with only 1 attempt taken until the last 2 minutes we can expect on average the same amount of trips (11.8) but with only 1 attempt worth the new 1.44 PPS. This results in around 17 points per game from the line. Accounting for normal rules in the last 2 minutes we get

Expected points = 46/48 * (17) + 2/48 * (17.5)

= 17.03

This is under an assumption that we have a uniform distribution rate of fouls in the first 46 minutes and the last two minutes. This only results in a 0.5 point reduction per game which spread to an 82 game season only accounts to 38.5 fewer points. Could this cause more intentional fouling late in games up until the last two minutes? The variance on these shots could cause very different outcomes in certain games but over larger samples should have little effect.

More substantial game swings happen in the last two minutes, which if the rule lasted all game would then cause a sizable effect on game outcomes. Essentially this rule will create a quicker game to watch by shaving off minutes and create more variance in free-throw shooting. However, the long-term impact will create marginal differences that won’t have a strong statistical impact on scoring.